Vectorial Poincaré beams

Polarization is one of the fundamental yet fascinating properties of transverse waves, as light waves, gravitational waves, and sound waves in solids, i.e. it plays an important part in optics, seismology, radio, and microwaves. Especially, topical technologies in the field of photonics as lasers itself, or optical fiber technology are impacted by polarization, resulting in growing attention with respect to the investigation and manipulation of this property of light.

Research regarding this property of light led to the finding of so called vectorial Poincaré beams. In contrast to scalar light fields showing a homogeneous distribution in polarization, these beams reveal different states of polarization within their transverse plane [Otte2015, Alpmann2016]. The contained states of polarization (SOPs) belong to single or multiple parts of the Poincaré sphere (a unit sphere used for the visualization of polarization spanned by Stokes parameters), causing the light fields name. Due to the distribution of SOPs, particular locations can occure where a specification of polarization vanishes within the spatially structured beam. In these polarization singularities the SOP itself (V-Point), its orientation (C-Point/ Line) or handedness (L-Point/ Line) is undefined [Otte2015, Alpmann2016]. The investigation of these light fields and their singularities belongs to the research area of Singular Optics. Furthermore, the Poincaré beam’s properties are of special interest with respect to their wide range of applications as e.g. optical trapping at the nanoscale, super resolution imaging and high-capacity information encoding.

In order to experimentally generate tailored Poincaré beams including polarization singularities of predefined kind, order, number and position, we employ a holographic technique based on a phase-only spatial light modulator combined with two wave plates [Otte2015, Alpmann2016]. This method enables a dynamic, spatially resolved modulation of polarization resulting in novel kinds of Poincaré beams.

We investigate linearly polarized vector beams, whose orientation vary regularly within the transverse plane, so that they can resemble flowers and spider webs [Otte2016]. Moreover, with our approach it is also possible to generate and analyze irregularly structured and even hybrid vector fields [Otte2016]. These novel kinds of Poincaré beams reveal the shape of deformed flowers/ webs and hybrids of flowers and webs (see Figure below), enabling the investigation of fundamental issues of singular optics. Beyond this, these fields pave the way to extended applications of tailored light fields.  

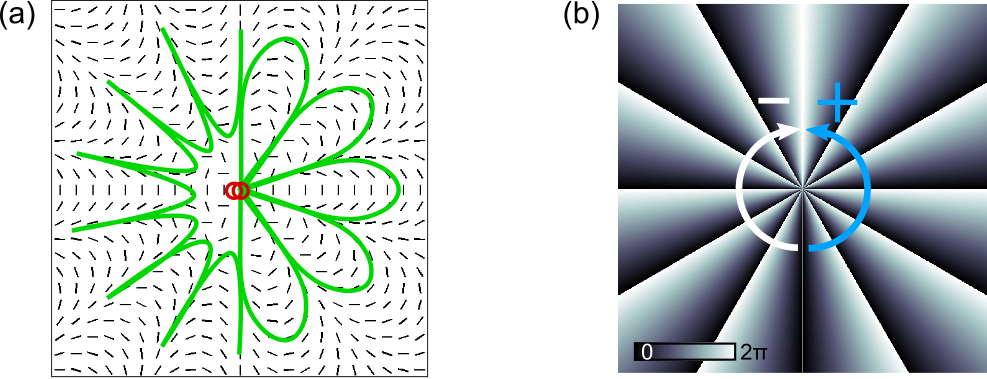
Vectorial hybrid structure of flower and spider web: (a) polarization distribution in the beam’s transverse plane superimposed by green flow lines of polarization vectors’ orientation, red: polarization singularities; (b) phase Φ_12=arctan⁡(S_2/S_1) of the complex Stokes field Σ_12=S_1+iS_2 (see [Otte2016])

Beside vector fields, ellipse fields represent another subset of Poincaré beams, containing linear as well as elliptical and circular SOPs. By our holographic method, we are also able to generate these kinds of beams revealing different singularities. We analyze created beams in the transverse plane, but also their longitudinal evolution. Furthermore, investigations in the image and Fourier plane are facilitated.

Our approach can be extendable to deal with more complex issues, like the combination of both, scalar light fields and vectorial Poincaré beams, by joint amplitude, phase and polarization modulation [Otte2015, Alpmann2016]. This method facilitates tailored light fields, which reveal  novel approaches for e.g. optical micromanipulation: a combinition of OAM (orbital angular momentum) and SAM (Spin angular momentum) structured beams can build customized energy landscapes and flow structures for optical tweezers.

References:

  • [Otte2015] Otte E, Schlickriede C, Alpmann C and Denz C 2015 “Complex light fields enter a new dimension: holographic modulation of polarization in addition to amplitude and phase” Proceedings of SPIE vol. 9379, 937908
  • [Otte2016] Otte E, Alpmann C, and Denz C 2016 “Higher-order polarization singularities in tailored vector beams” Journal of Optics 18 074012
  • [Alpmann2016] Alpmann C, Schlickriede C, Otte E and Denz C 2016 “Dynamic modulation of Poincaré beams” submitted