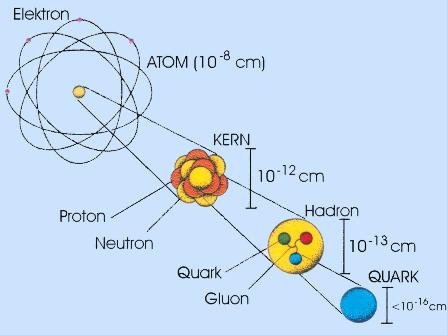
The last decades of research have shown that the known matter consists of a relatively small number of constituents. On the one hand there are the quarks, of which the atomic nuclei are made of. On the other hand there are the leptons, among which there are the electrons which make up the atomic shells.
Responsible for the interactions among those elementary particles are four different types of forces. Besides the gravitational force, which does not play an important role in the atomic regime, these are the electromagnetic force, the so-called weak and the strong interaction. The last two of which are responsible for processes in the domain of atomic nuclei and elementary particles, e. g. for radioactivity and nuclear fusion.
The fundamental particles and their interactions (except gravity) are theoretically described by the “Standard Model” of elementary particle physics. It has been formulated in the seventies of the last century and is nearly completely in accordance with countless numbers of experimental observations of particle physics. The Standard Model respects the principles of quantum theory and special relativity. It is an example for a “quantum field theory”.
In the Standard Model, forces between matter particles are mediated by several carriers. One example are the “field quanta” of the electromagnetic force field, that are identical to the light quanta, the photons.
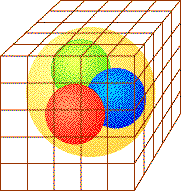
In our workgroup we deal with various questions from the realm of quantum field theory. There are theoretical approaches for physics beyond the Standard Model, that could reveal themselves at very high energies. These approaches make use of the concept of supersymmetry, a symmetry which extends the usual space-time symmetry. Among other things we investigate basic features of supersymmetric theories. Some calculations can be done with paper and pencil. Many question however cannot be solved this way, but require the application of numerical methods. Therefore we also perform extensive calculations on supercomputers. In these calculations it is necessary to replace the continuum of space-time by a lattice. By this means one deals with a finite number of variables, that can be handled numerically. The calculations of physical quantities are very complex and are done using statistical methods.
Another area of our research comprises the theory of the strong interactions of the elementary particles. These are contained in the Standard Model in the form of the so-called Quantum Chromodynamics. Due to the strength of the interactions between quarks, common approximation methods fail as they require sufficiently small couplings. Thus, to calculate properties of strongly interacting elementary particles computer methods are also used, in which Quantum Chromodynamics is put onto a lattice. Then masses and other characteristica of particles can be calculated. The outcomes of such calculations are very important for comparison with experimental results.
When examining theories on a lattice, the lattices have to be fine enough to be able to resolve the details of the particles; on the other hand the lattice has to be large enough to contain a particle as a whole. These demands result in a great need for computer performance.
For the calculations we use computational power that is provided to us on parallelized supercomputers of the Neumann-Institut for Computing (NIC) in Jülich and of DESY-Zeuthen.
Another part of our activities does not have to do with elementary particles. Remarkably also certain phenomena in the area of thermodynamics, the physics of heat, can be described by means of quantum field theory. This is true especially for phase transitions like vaporization, melting and condensation. In our workgroup we make use of this connection in order to obtain result about thermodynamical systems via methodes of quantum field theory.
As an example, the behavior of interfaces may be mentioned. Numerous systems possess interfaces that separate different phases, for instance between gas and liquid or between different liquids. Increasing the temperature, it might happen that an interface dissolves and that formerly seperated phases mix. The way the interface vanishes and the typical properties of the interface, that are also experimentally accessible, can be described and calculated by means of quantum field theory.
Phase transitions, as for instance condensation of vapour, can begin through nucleation. The condensation nuclei form droplets which will subsequently expand. The mechanism of droplet formation and the rate of nucleation during phase transitions are studied by us by means of quantum field theory.

