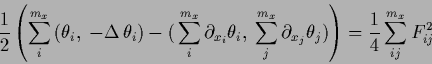Next: Invariant determinants
Up: Adapting prior covariances
Previous: Automatic relevance detection
Contents
Local masses and gauge theories
The Bayesian analog of a mass term in quantum field theory
is a term
proportional to the identity matrix 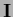 in the inverse prior covariance
in the inverse prior covariance  .
Consider, for example,
.
Consider, for example,
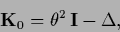 |
(464) |
with 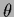 real (so that
real (so that 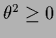 )
representing a mass parameter.
For large masses
)
representing a mass parameter.
For large masses 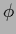 tends to copy the template
tends to copy the template 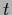 locally,
and longer range effects of data points following from
smoothness requirements become less important.
A constant mass can be replaced by a mass function
locally,
and longer range effects of data points following from
smoothness requirements become less important.
A constant mass can be replaced by a mass function 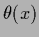 .
This allows to adapt locally that interplay between ``template copying''
and smoothness related influence of training data.
As hyperprior, one may
use a smoothness constraint on the mass function
.
This allows to adapt locally that interplay between ``template copying''
and smoothness related influence of training data.
As hyperprior, one may
use a smoothness constraint on the mass function 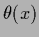 ,
e.g.,
,
e.g.,
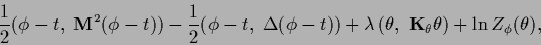 |
(465) |
where 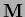 denotes the diagonal mass operator
with diagonal elements
denotes the diagonal mass operator
with diagonal elements 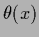 .
.
Functional hyperparameters
like 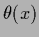 represent,
in the language of physicists,
additional fields entering the problem
(see also Sect. 5.6).
There are similarities for example to gauge fields in physics.
In particular, a gauge theory-like formalism can be constructed
by decomposing
represent,
in the language of physicists,
additional fields entering the problem
(see also Sect. 5.6).
There are similarities for example to gauge fields in physics.
In particular, a gauge theory-like formalism can be constructed
by decomposing 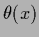 =
=
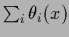 ,
so that the inverse covariance
,
so that the inverse covariance
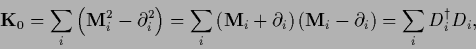 |
(466) |
can be expressed in terms of a ``covariant derivative''
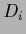 =
=
 .
Next, one may choose as hyperprior for
.
Next, one may choose as hyperprior for 
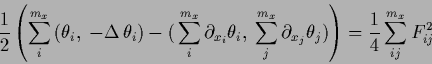 |
(467) |
which can be expressed in terms of
a ``field strength tensor'' (for Abelian fields),
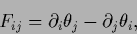 |
(468) |
like, for example,
the Maxwell tensor in quantum electrodynamics.
(To relate this, as in electrodynamics,
to a local 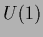 gauge symmetry
gauge symmetry
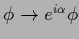 one can consider complex functions
one can consider complex functions 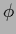 ,
with the restriction that their phase cannot be measured.)
Notice, that, due to the interpretation of the prior as
product
,
with the restriction that their phase cannot be measured.)
Notice, that, due to the interpretation of the prior as
product
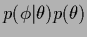 , an additional
, an additional 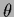 -dependent
normalization term
-dependent
normalization term
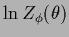 enters the energy functional.
Such a term is not present in quantum field theory,
where one relates the prior functional directly to
enters the energy functional.
Such a term is not present in quantum field theory,
where one relates the prior functional directly to
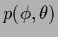 , so the norm is independent of
, so the norm is independent of 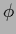 and
and 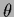 .
.




Next: Invariant determinants
Up: Adapting prior covariances
Previous: Automatic relevance detection
Contents
Joerg_Lemm
2001-01-21
![]() in the inverse prior covariance
in the inverse prior covariance ![]() .
Consider, for example,
.
Consider, for example,
![]() represent,
in the language of physicists,
additional fields entering the problem
(see also Sect. 5.6).
There are similarities for example to gauge fields in physics.
In particular, a gauge theory-like formalism can be constructed
by decomposing
represent,
in the language of physicists,
additional fields entering the problem
(see also Sect. 5.6).
There are similarities for example to gauge fields in physics.
In particular, a gauge theory-like formalism can be constructed
by decomposing ![]() =
=
![]() ,
so that the inverse covariance
,
so that the inverse covariance